Simplicity, Cones, and Naturalism

I think I may have figured out something helpful by reflecting on a conversation with Graham Oppy about the root of reality. Seeing this may help our views come closer together. In my first YouTube conversation with Graham, I brought the tools of (i) simplicity, (ii) explanatory depth, and (iii) uniformity. I thought Graham would especially appreciate tools (i) and (ii). I was surprised, then, when Graham said he was thinking of things very differently. Since then, I've pondered the potential root of the difference. This morning, I got a picture in my mind that I think may explain the difference and perhaps even remove it. I want to share the picture with you because it may help unify different perspectives or perhaps help others in thinking about their view.

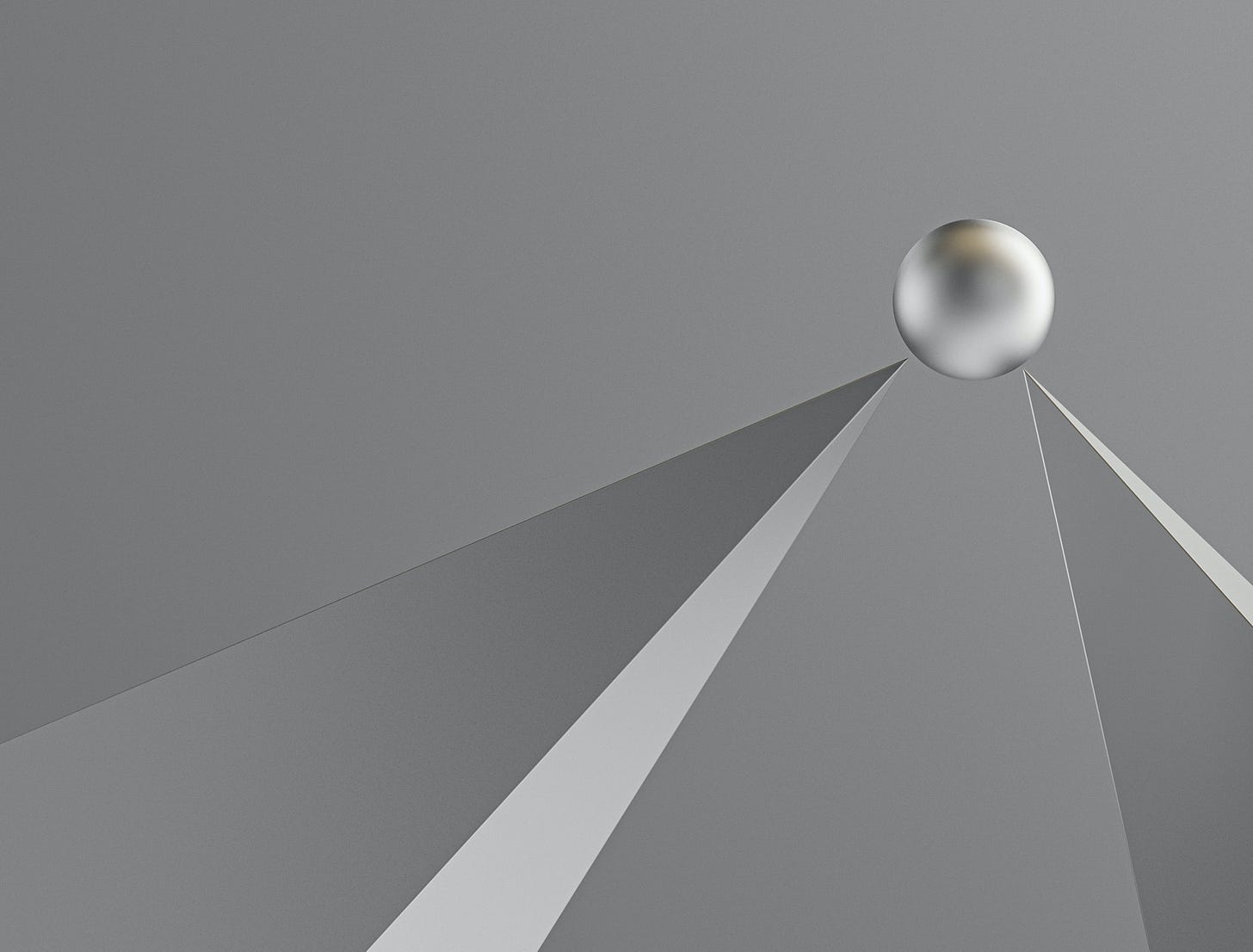
So the picture is of a cone that proceeds from a person's direct experiences (all of them). This cone represents a sequence of causal explanations of one's experiences going back to an initial causal segment, if there is an initial segment; if there is no initial segment, then the cone is infinite. You can think of the cone from your own perspective as a representing the sequence of explanations of your total experiences.

Different theories of the world imply different shapes of the cone. If explanations get increasingly complex (in terms of the number of primitive terms/components required to express them), then the cone widens; if the explanations get increasingly simple, then the cone narrows. If the world came into existence 10 minutes ago, then the cone is shorter than if the world is older than 10 minutes. If the initial explanation is complex, then the cone is bounded by a larger edge than if the initial explanation is simpler.

Now let's call the things you know by direct experience your "direct data." My question is, what does the cone (chain of explanations) leading to your direct data look like?

Here's one answer: the cone extends through a short sequence and abruptly ends with a flat edge, representing 10 minutes ago. Surely, that's not probable. But why not?

Consider, first, some motivation for the short-cone hypothesis. It has a certain simplicity advantage over longer cones. After all, longer cones add more explanations, and these additional explanations go beyond what's needed to explain everything you know by direct experience. Why posit explanations beyond necessity?

We might reply that, despite the simplicity advantage, the short cone lacks explanatory depth. In particular, it multiplies brute facts at the base of the cone. Explanatory depth is worth the cost of complexity, we might think.

Not so fast. A defender of the short cone can reply that the base of the cone isn't brute but is instead explained by its necessity (i.e., the temporal edge of the world ten minutes ago couldn't have not existed). So brute facts aren't actually multiplied. In fact, each necessity can be explained by the necessity of its being necessary—giving us infinite explanatory depth! So the short-cone enjoys explanatory depth and simplicity. (And it successfully predicts all your direct data.)

Trading complexity for explanatory depth doesn't help, then. The root problem lies elsewhere. Where?

A more fundamental problem with the short cone, I think, is that it lacks a unifying explanation. Its front edge has many disparate parts (e.g., all your experiences and memories appearing from nothing). A unifying explanation that successfully predicts those many parts (e.g., in terms of laws of physics, a causal order, etc.) explains more with less. That's an advantage. The result, in effect, simplifies the end of the cone.

This picture relates to my conversation with Graham about the root of reality. I was proposing to simplify the end of the cone. However, he was seeing (rightly) that my proposal also extended the cone's length by adding an explanation. In that sense, I was indeed adding complexity.

Indeed, it may sound really weird to hear me propose that I'm shaving complexity. After all, I'm the one positing something in addition to what "we" know (from our best science). I'm the one extending the cone. Sure, I might try to argue that it's worth trading complexity for explanatory depth, but certainly I'm not offering a simpler theory by adding something to the cone!

The picture of the cone helps clarify our perspectives. I heartily agree that a theory that extends the cone adds something. In that sense, it is more complex. Furthermore, the addition is not automatically justified just by its greater explanatory depth; after all, even a 10-minute-old cone can enjoy infinite explanatory depth. Rather, the addition is justified by its unifying power. A cone with a simpler end has more unifying power than a cone with a more complex end.

It's important to realize that your direct data is all the direct data you have. Everything else is an inference, such as an inference to the best explanation of your direct data. A total theory of the world that includes all and only your direct data is of course simpler than a theory that includes in addition some explanation of your direct data. In that sense, you always get more simplicity by stopping explanations shorter. A cost of going too short, though, is a lack of unification. A question, then, for everyone is, how short is too short?

Whatever you think about ultimate reality, you can use the cone picture as a tool to think about large scale theories. Consider what general shape makes the most sense of your all your direct data (including your experience of testimonies from physicists). Does the cone extend outward infinitely, come to a relatively simple end, come to a relatively complex end, etc.? What are the advantages/disadvantages of different cone shapes?

While different people have different views about the contents of the cone (e.g., not many people include my cat in their cone), perhaps we can come to a more unified vision of the general shape of the cone.

I wish to thank Joe Schmid, Kyle Alander, Micah Edvenson, and of course, Graham Oppy for seeds and nutrients for these thoughts. Any mistakes or deficiencies are due to me.